Overview of Trigonometric Ratios for Specific Angles
Trigonometric ratios for specific angles—,
,
,
, and
—are essential in trigonometry. These angles, often referred to as standard angles, have predefined trigonometric values that simplify calculations. Memorizing these ratios allows for efficient problem-solving in trigonometry without requiring a calculator.
Trigonometric ratios for these angles are derived from geometric principles, such as properties of equilateral triangles, isosceles triangles, and the Pythagorean theorem.
Why These Angles Are Considered Standard
- Symmetry and Simplicity:
- These angles appear naturally in symmetrical geometric shapes like equilateral and isosceles right triangles.
- The trigonometric ratios for these angles follow specific patterns, making them easy to remember and compute.
- Frequent Occurrence in Applications:
- These angles commonly occur in real-world problems, such as calculating heights, distances, slopes, and angles of elevation or depression.
- They are also critical in fields like physics (wave motion, projectile motion) and engineering (design and architecture).
- Geometric Foundation:
and
: Derived from dividing an equilateral triangle into two right triangles.
: Found in isosceles right triangles (
).
and
: Represent extreme cases where the angle collapses to a line or a vertical, respectively.
Trigonometric Ratios for Standard Angles
Key Angles
The key angles in trigonometry are:
,
,
,
, and
.
These angles are used frequently in solving trigonometric problems due to their predictable and simplified trigonometric ratios.
Table of Trigonometric Ratios
Explanation of Table
and
:
- These values are derived from the geometric properties of right triangles.
and
are complementary for these angles:
.
:
.
- Undefined for
, as
.
- Reciprocal Ratios:
.
.
.
Derivation of Ratios for Specific Angles
For 
- Consider an Isosceles Right Triangle:
In atriangle:
- The two legs are equal, and let their length be
.
- The hypotenuse is calculated using the Pythagorean theorem:
- The two legs are equal, and let their length be
- Trigonometric Ratios for
: Using the definitions of trigonometric ratios:
.
.
.
For  and
and 
- Consider an Equilateral Triangle:
- An equilateral triangle is split into two right triangles, dividing the angles as
.
- Let the side of the equilateral triangle be
.
- After splitting:
- The hypotenuse =
.
- The side opposite
=
.
- The side adjacent to
=
.
- The hypotenuse =
- An equilateral triangle is split into two right triangles, dividing the angles as
- Trigonometric Ratios for
: Using the definitions:
.
.
.
- Trigonometric Ratios for
: Using the same triangle:
.
.
.
Summary of Ratios
- For
:
.
- For
:
.
- For
:
.
Applications of Trigonometric Ratios for Standard Angles
Practical Uses
- Solving Geometry Problems Involving Known Angles
- Identifying Unknown Sides:
Trigonometric ratios for standard angles help determine unknown side lengths of triangles when at least one side and an angle are known.- Example: In a
triangle, the sides are in the ratio
. Using this, you can quickly calculate side lengths.
- Example: In a
- Verifying Shapes:
Standard angles are used to analyze and verify geometric shapes like squares, equilateral triangles, and isosceles triangles.
- Identifying Unknown Sides:
- Simplifying Calculations in Height and Distance Problems
- Height of an Object:
Standard angles are used to find the height of an object using the angle of elevation and a known horizontal distance.- Example:
If the angle of elevation to the top of a building isand the distance from the base is
, then:
.
- Example:
- Distance Between Two Points:
By using the angle of depression and a known height, the horizontal distance can be calculated.
- Height of an Object:
- Applications in Physics
- Wave Motion:
Trigonometric functions, including standard angles, describe the oscillatory behavior of sound and light waves.
Example: A wave’s displacement can be expressed as, where
is evaluated at standard angles.
- Projectile Motion:
The range and height of projectiles depend on trigonometric functions of the launch angle:- Range:
- Maximum Height:
Here, standard angles likeare used for maximizing range.
- Range:
- Resolving Forces:
In mechanics, forces are often broken down into components along the x and y axes usingand
.
- Wave Motion:
Tips for Memorization
Use Patterns for and
The trigonometric values of and
for the standard angles (
,
,
,
,
) follow predictable patterns that can be memorized easily:
- For
:
The values increase fromto
in a symmetrical progression:
- For
:
The values decrease fromto
, mirroring
:
Derive from
- To find
for any standard angle:
- Example:
- For
:
- For
:
- For
Use Mnemonics
To memorize trigonometric values:
- For
:
Think of the pattern “0, 1/2, 1/√2, √3/2, 1.” - For
:
Reverse thepattern: “1, √3/2, 1/√2, 1/2, 0.”
Practice Reciprocals
Example:
Related Topics
- Criteria for Similarity of Triangles
- Trigonometric Ratios
- nth Term of an AP
- Similarity of Triangles
- Mensuration
- Solution of a Quadratic Equation by Factorisation
- Introduction to Triangles
- Congruence of Triangles
- Some applications Of Trigonometry
- Sum of First n Terms of an AP
- Roman Numerals
- Coordinate Geometry Distance Formula
- Area of Triangle
- Real Numbers
- Trigonometric Identities



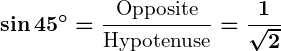 .
. .
. .
.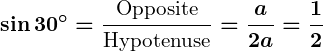 .
. .
.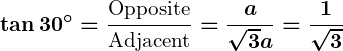 .
.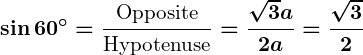 .
. .
. .
.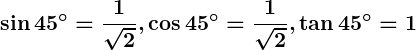 .
. .
.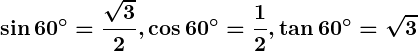 .
.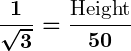
 .
.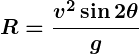
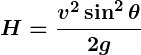
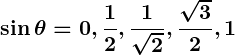
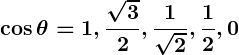
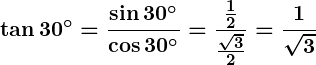







Get Social