What is Coulomb’s Law?
Coulomb’s Law, a cornerstone in the study of electromagnetism, describes the force between two point charges. Established by French physicist Charles-Augustin de Coulomb in 1785, this law states that the force between two charged objects is directly proportional to the product of their charges and inversely proportional to the square of the distance between them. This fundamental interaction underpins much of our understanding of electrical forces in physics, influencing everything from atomic interactions to electrical engineering.
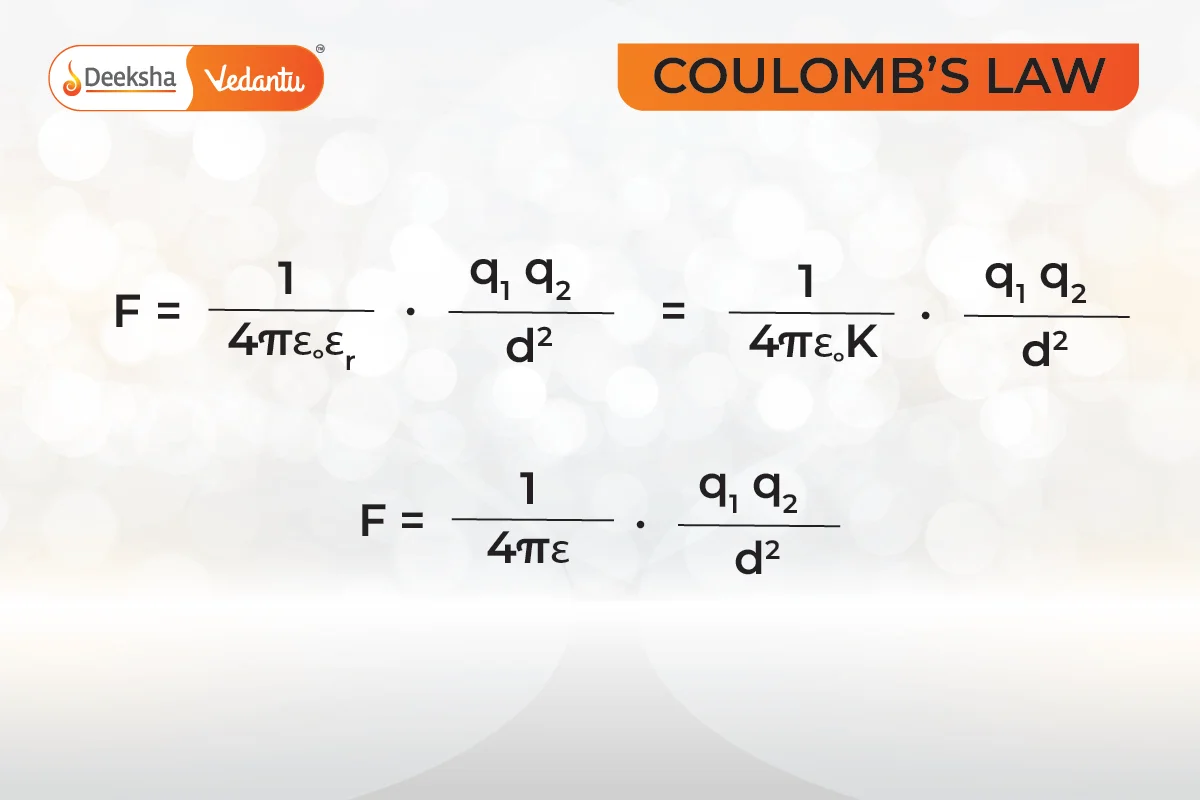
In short,
where:
is absolute permittivity.
or
is the relative permittivity or specific inductive capacity.
is the permittivity of free space.
or
is also called the dielectric constant of the medium in which the two charges are placed.
Mathematical Formulation of Coulomb’s Law
The law is mathematically expressed as:
where:
is the magnitude of the electrostatic force between the two charges.
and
are the amounts of the charges.
is the distance between the centers of the two charges.
is Coulomb’s constant
, which depends on the medium in which the charges reside.
Vector Form of Coulomb’s Law
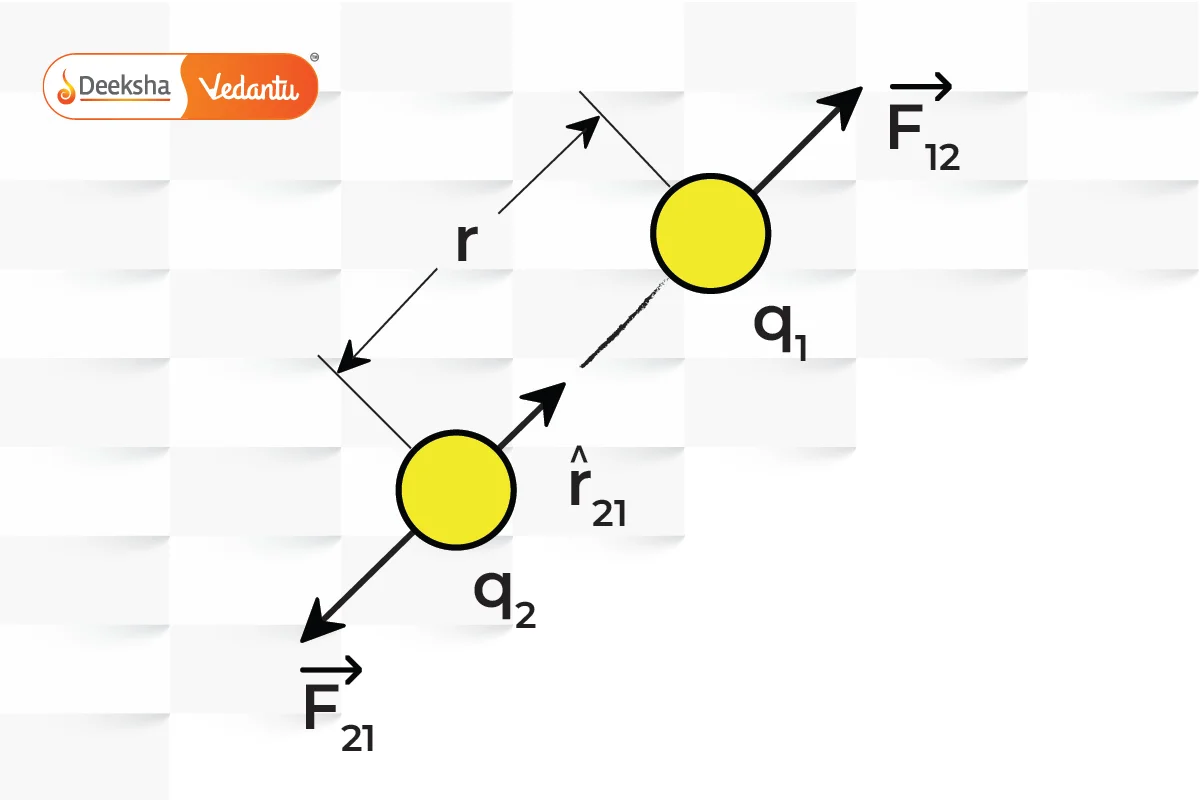
In vector form, Coulomb’s Law helps to determine the direction of the force along with its magnitude. If you consider two charges, and
, positioned at points
and
, the force
exerted on
by
is given by:
This vector formulation indicates that the force acts along the line joining the charges, being repulsive if the charges are of like sign and attractive if they are of opposite sign.
Applications and Limitations of Coulomb’s Law
Coulomb’s Law is pivotal in fields ranging from electrostatics to quantum mechanics. It is used to calculate the forces between charged particles, which is essential in designing electric circuits, understanding chemical bonding, and even in technological applications like photocopiers and inkjet printers.
However, Coulomb’s Law has its limitations:
- It applies only to stationary charges.
- The law assumes point charges, which is rarely the case in practical scenarios.
- The effects of medium between charges are simplified, not accounting for complex interactions in non-uniform materials.
Key Points on Coulomb’s Law
Here, we delve deeper into specific scenarios that demonstrate how Coulomb’s Law applies in various situations.
1. Consistency of Force Across Different Media:
If the electrostatic force between two charges remains constant despite being placed in different media, it implies a direct relationship between the relative permittivity of the medium () and the square of the separation distance (
). Mathematically, this can be expressed as
. This principle helps in understanding how materials affect electromagnetic forces.
2. Force Equivalence in Different Conditions:
When the force between two charges in a vacuum is equal to that in another medium at different separation distances, the relationship (where
is the original distance in vacuum) holds. This equation helps in calculating the effective permittivity of the medium based on changes in distance.
3. Charge Redistribution Among Conductors:
When two conductors carrying charges and
are brought into contact and then separated:
- The charges redistribute equally, with each conductor having
.
- If one charge is negative (say
), then each conductor ends up with
.
- The charges redistribute equally, with each conductor having
4. Redistribution in Spherical Conductors:
For spherical conductors with radii and
and charges
and
, upon contact and separation, the charge on each becomes:
This demonstrates how geometric proportions influence charge distribution.
5. Force Adjustment Post-Contact:
If two identical charged conductors are touched together and then separated back to a distance , the new force
between them, assuming one charge becomes negative, is
.
This illustrates how contact affects the electrical interaction due to redistribution of charges.
6. Comparative Force Analysis:
The force comparisons provide insight into the vast differences between electrical and gravitational forces:
- Between two electrons:
- Between two electrons:
- Between two protons:
- Between two protons:
- Between a proton and an electron:
- Between a proton and an electron:
7. Light Velocity and Electromagnetic Constants:
The relationship between the speed of light (), the permeability of free space (
), and the permittivity of free space (
) is given by
. This fundamental relationship ties together electromagnetic theory with the physical constants of the vacuum.
FAQs
- Coulomb’s constant (k) determines the magnitude of the electrostatic force in a given medium. It varies with the permittivity of the medium, being higher in a vacuum and lower in other media.
- Coulomb’s Law not only helps in understanding the natural phenomena related to charges but also serves as a building block for more complex theories in electromagnetism and quantum physics, highlighting the interconnectivity of science across various domains.
Coulomb’s Law can be applied to spheres if they are considered as point charges located at their centers, provided the distance between them is much greater than their radii.
The force is inversely proportional to the square of the distance between the charges. As the distance increases, the force decreases rapidly.
Yes, Coulomb’s Law applies to both attractive and repulsive forces. The force is attractive between opposite charges and repulsive between like charges.
Coulomb’s Law is used to calculate the electrostatic force between two point charges. It is fundamental in fields such as chemistry, physics, and electrical engineering.
Related Topics
- JEE Main Marks vs Rank 2024
- Correlation Coefficient
- Chemical Bonding
- Binomial Theorem
- JEE Advanced Marks vs Ranks 2024
- Rank of a Matrix and Some Special Matrices
- JEE Main Syllabus 2025
- Electromagnetic Spectrum
- Transformer
- Young’s Double Slit Experiment
- Redox Reactions
- Heisenberg Uncertainty Principle
- Basic Logic Gates
- Simple Harmonic Motion (SHM)
- Normality











Get Social