Kirchhoff’s circuit laws are fundamental principles used in electrical engineering to analyze circuits. These laws, known as Kirchhoff’s Current Law (KCL) and Kirchhoff’s Voltage Law (KVL), help us understand the behavior of electrical circuits and predict how current and voltage will distribute through various components.
History about Gustav Robert Kirchhoff
Gustav Robert Kirchhoff was a German physicist born on March 12, 1824, in Königsberg, Prussia. His pioneering work in the conduction of electricity led to the formulation of Kirchhoff’s Laws of Closed Electric Circuits in 1845. These laws have become essential in the field of electrical engineering. Kirchhoff also contributed significantly to spectroscopy and blackbody radiation studies. He was the first to verify that electrical impulses travel at the speed of light.

What Are Kirchhoff’s Laws?
In 1845, Gustav Kirchhoff introduced two fundamental laws that apply to electrical circuits: Kirchhoff’s Current Law (KCL) and Kirchhoff’s Voltage Law (KVL).
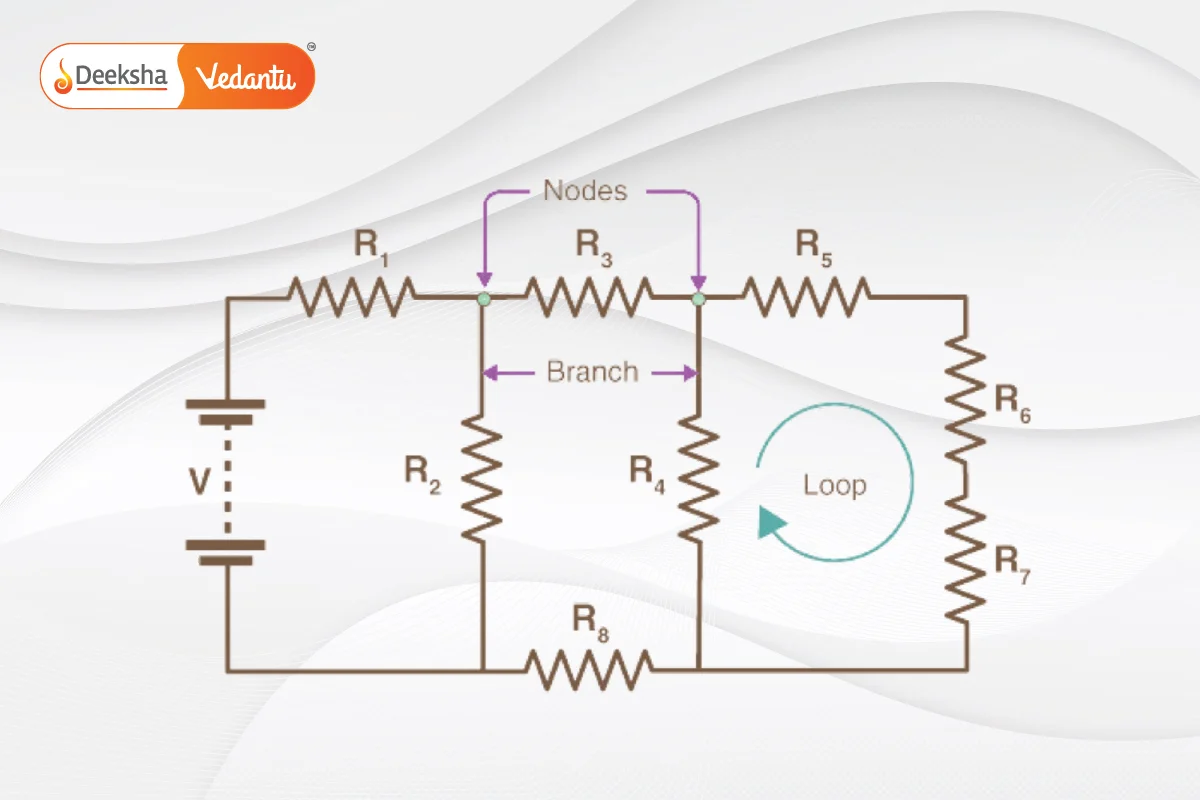
Kirchhoff’s Current Law (KCL)
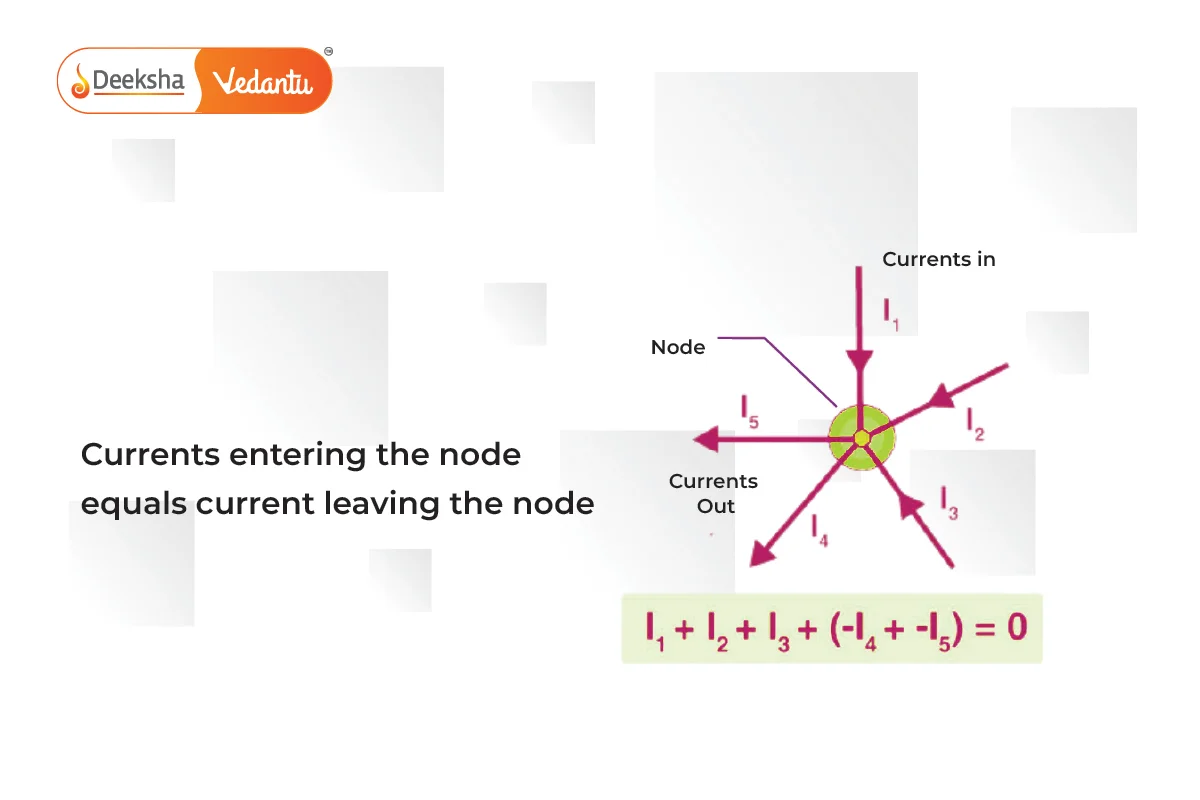
Kirchhoff’s Current Law, also known as Kirchhoff’s First Law or Kirchhoff’s Junction Rule, states:
“The total current entering a junction (or node) is equal to the total current leaving the junction.”
This law is based on the conservation of charge, meaning that charge cannot be created or destroyed. In a junction, the sum of currents flowing into the node equals the sum of currents flowing out of the node. Mathematically, it can be expressed as:
I1 + I2 + I3 = I4 + I5
where I1, I2, and I3 are the currents entering the node, and I4 and I5 are the currents leaving the node.
Kirchhoff’s Voltage Law (KVL)
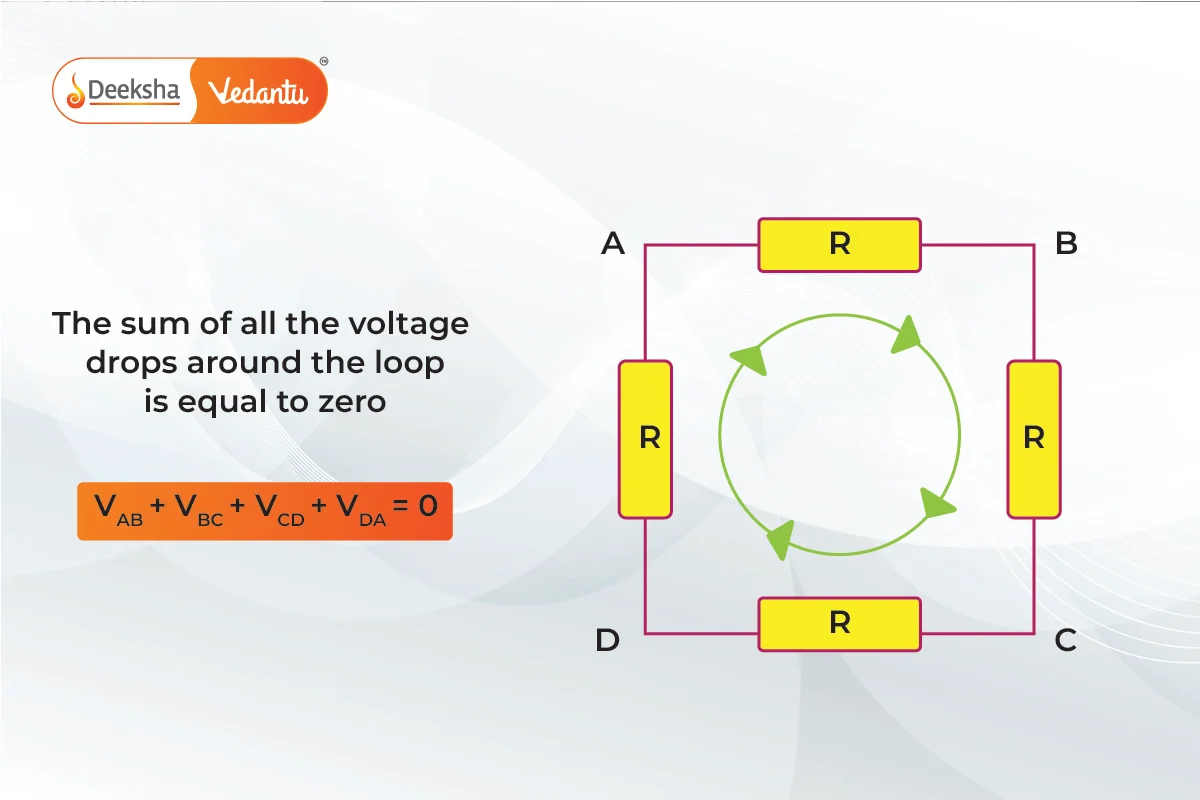
Kirchhoff’s Voltage Law, also known as Kirchhoff’s Second Law or Kirchhoff’s Loop Rule, states:
“The sum of the electrical potential differences (voltage) around any closed circuit loop is zero.”
This law is based on the conservation of energy. It means that the total amount of energy gained per unit charge is equal to the total amount of energy lost per unit charge around a loop. Mathematically, it can be expressed as:
V1 + V2 + V3 = 0
where V1, V2 , and V3 are the voltages around a closed loop.
Applying Kirchhoff’s Laws
Kirchhoff’s Current Law (KCL)
To apply KCL, follow these steps:
- Identify all the junctions in the circuit.
- Assign current directions for each branch connected to the junction.
- Write the KCL equation for each junction, summing the currents entering and leaving the junction and setting the sum equal to zero.
Example:
If I1, I2, and I3 are currents entering a node and I4 and I5 are currents leaving the node, then:
I1 + I2 + I3 = I4 + I5
Kirchhoff’s Voltage Law (KVL)
To apply KVL, follow these steps:
- Identify the closed loops in the circuit.
- Choose a direction to traverse the loop (clockwise or counterclockwise).
- Sum the voltages around the loop, taking into account the sign of each voltage drop (positive for voltage gain and negative for voltage drop), and set the sum equal to zero.
Example:
For a loop with voltages V1, V2, and V3 :
V1 – V2 – V3 = 0
FAQs
Yes, Kirchhoff’s Laws can be applied to both AC and DC circuits to analyze current and voltage distributions.
The negative sign in KVL indicates the direction of voltage drops and gains around the loop, ensuring the conservation of energy.
To apply KVL, identify closed loops in the circuit, choose a direction to traverse the loop, sum the voltages around the loop considering the sign of each voltage drop, and set the sum equal to zero.
To apply KCL, identify all junctions in the circuit, assign current directions, write the KCL equation for each junction, and sum the currents entering and leaving the junction to set the sum equal to zero.
Kirchhoff’s Laws are essential for analyzing and understanding electrical circuits, allowing for the calculation of current and voltage in complex networks.
Kirchhoff’s Laws were discovered by Gustav Robert Kirchhoff, a German physicist, in 1845.
Kirchhoff’s Laws consist of Kirchhoff’s Current Law (KCL) and Kirchhoff’s Voltage Law (KVL). KCL states that the total current entering a junction equals the total current leaving it, while KVL states that the sum of all voltages around a closed loop is zero.
Related Topics
- Faraday’s Law
- Pressure
- Magnetic Field Due To A Current – Carrying Conductor
- Fleming’s Left-Hand Rule and Right-Hand Rule
- Circuit Diagram
- Defects Of Vision And Their Correction
- Full Wave Rectifier
- Resistance Of A System Of Resistors
- Magnetic Field And Field Lines
- Electric Current And Circuit
- Domestic Electric Circuits
- Projectile Motion
- Laws of Motion
- Ohm’s Law
- Scattering Of Light












Get Social