Introduction
Spherical mirrors are mirrors with curved surfaces that are a part of a sphere. Unlike plane mirrors that have flat surfaces, spherical mirrors have surfaces that can be either curved inward or outward. These mirrors obey the laws of reflection and are commonly used in daily life, from vehicle mirrors to telescopes.
What are Spherical Mirrors?
A spherical mirror is a mirror that has the shape of a part of a hollow sphere. The reflective surface of the mirror can either be curved inward or outward. There are two types of spherical mirrors:
- Concave Mirrors (Converging mirrors): These mirrors have a reflective surface that is curved inward, like the inside of a bowl.
- Convex Mirrors (Diverging mirrors): These mirrors have a reflective surface that is curved outward, like the outside of a balloon.
Examples of Spherical Mirrors:
- Concave mirrors: Used in torches, car headlights, shaving mirrors.
- Convex mirrors: Used as rear-view mirrors in vehicles, as they give a wider field of view.
Terminology of Spherical Mirrors
To understand spherical mirrors, it’s essential to familiarize yourself with the key terms associated with them:
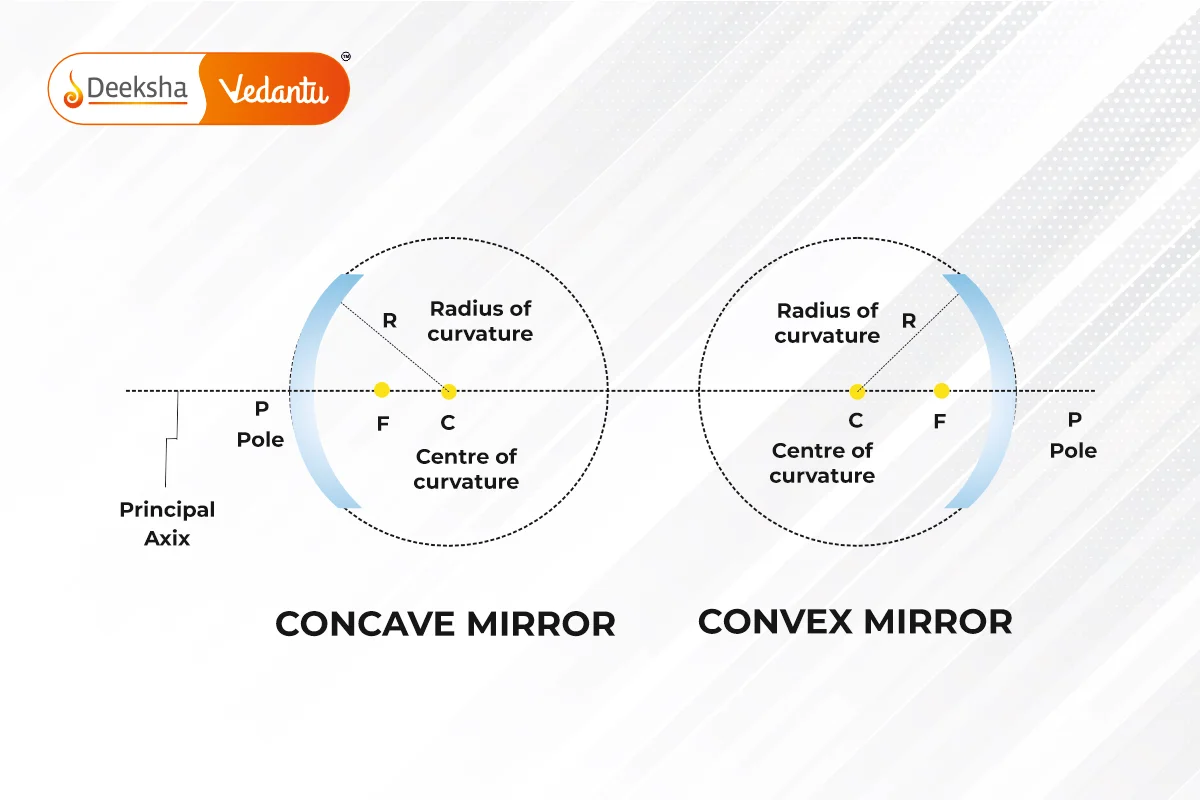
- Pole (P): The center of the reflecting surface of the mirror.
- Center of Curvature (C): The center of the sphere from which the mirror is derived. It lies outside the mirror for both concave and convex mirrors.
- Radius of Curvature (R): The radius of the sphere of which the mirror is a part. It is the distance between the pole and the center of curvature.
- Principal Axis: An imaginary line that passes through the center of curvature and the pole of the mirror.
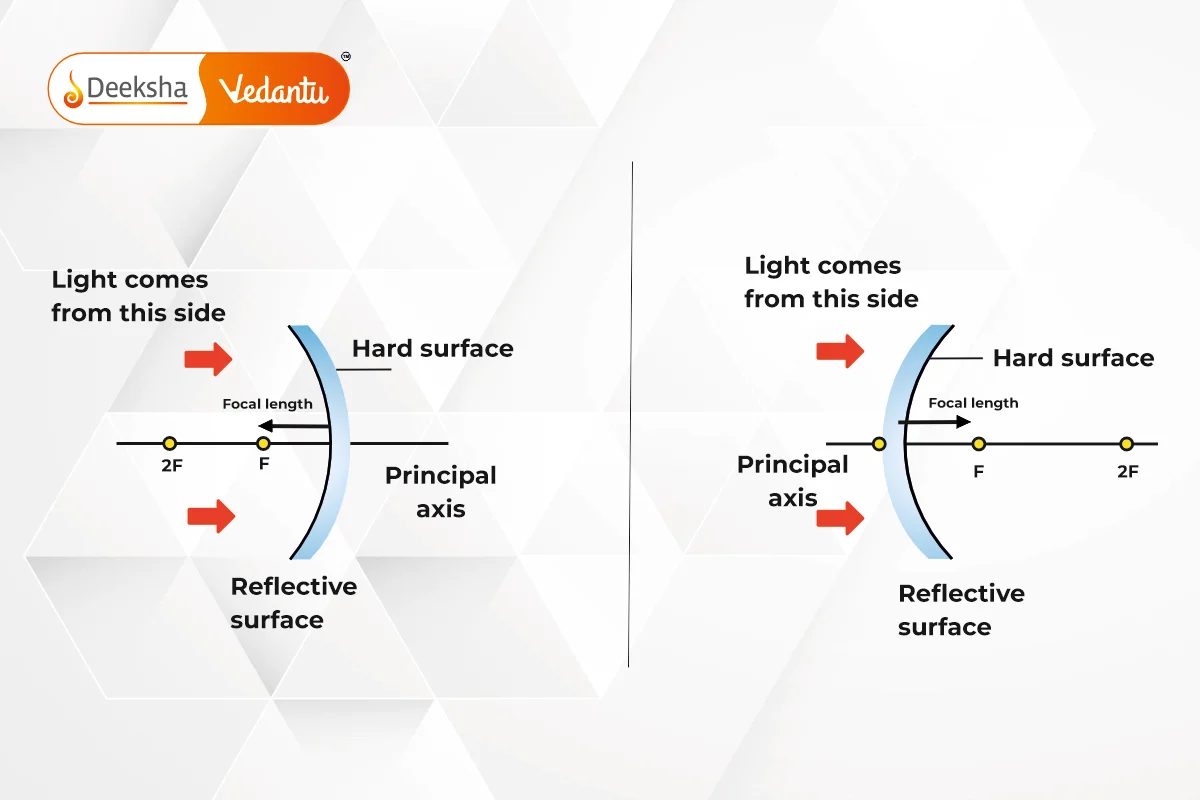
- Principal Focus (F): The point on the principal axis where light rays parallel to the principal axis converge (in concave mirrors) or appear to diverge (in convex mirrors) after reflection.
- Focal Length (f): The distance between the pole (
) and the focus (
) of the mirror. It is related to the radius of curvature as:![\[\displaystyle \boldsymbol{f = \frac{R}{2}}\]](data:image/svg+xml,%3Csvg%20xmlns%3D%27http%3A%2F%2Fwww.w3.org%2F2000%2Fsvg%27%20width%3D%2767%27%20height%3D%2747%27%20viewBox%3D%270%200%2067%2047%27%3E%3Crect%20width%3D%2767%27%20height%3D%2747%27%20fill-opacity%3D%220%22%2F%3E%3C%2Fsvg%3E "Rendered by QuickLaTeX.com")
) and the focus (
) of the mirror. It is related to the radius of curvature as:![\[\displaystyle \boldsymbol{f = \frac{R}{2}}\]](https://deekshalearning.com/wp-content/ql-cache/quicklatex.com-e298d72e9b489cd00674f2f9ecc9a637_l3.png "Rendered by QuickLaTeX.com")
Types of Spherical Mirrors
Concave Mirrors:
- Definition: A concave mirror has an inward-curved reflecting surface. It is also known as a converging mirror because it converges parallel rays of light that hit it.
- Image Formation: The image formed by a concave mirror can be real or virtual, depending on the position of the object. The nature, size, and position of the image vary depending on the distance between the object and the mirror.
Convex Mirrors:
- Definition: A convex mirror has an outward-curved reflecting surface. It is also known as a diverging mirror because it spreads out parallel rays of light that hit it.
- Image Formation: A convex mirror always forms a virtual, erect, and diminished image. The image appears smaller than the object and is located behind the mirror, making it ideal for use in vehicle mirrors.
Image Formation by Spherical Mirrors
The nature and size of the image formed by spherical mirrors depend on the position of the object relative to the mirror. For concave mirrors, the image can be real and inverted or virtual and erect, while for convex mirrors, the image is always virtual, erect, and smaller than the object.
Image Formation by Concave Mirrors:
The image formed by a concave mirror depends on the position of the object in relation to the principal focus (F) and the center of curvature (C).
| Object Position | Image Position | Nature of Image | Size of Image |
| At infinity | At Focus (F) | Real, inverted | Highly diminished |
| Beyond C | Between F and C | Real, inverted | Diminished |
| At C | At C | Real, inverted | Same size |
| Between C and F | Beyond C | Real, inverted | Enlarged |
| At F | At infinity | Real, inverted | Highly enlarged |
| Between F and P | Behind the mirror | Virtual, erect | Enlarged |
Image Formation by Convex Mirrors:
The image formed by a convex mirror is always virtual, erect, and smaller than the object.
| Object Position | Image Position | Nature of Image | Size of Image |
| At infinity | At F behind the mirror | Virtual, erect | Highly diminished |
| Between infinity and P | Between F and P behind mirror | Virtual, erect | Diminished |
Ray Diagrams for Spherical Mirrors
Concave Mirrors: To locate the image formed by concave mirrors, we use the following rays:
- A ray parallel to the principal axis reflects through the principal focus (F).
- A ray passing through the center of curvature (C) reflects back along the same path.
- A ray passing through the focus (F) reflects parallel to the principal axis.
Convex Mirrors: For convex mirrors:
- A ray parallel to the principal axis appears to diverge from the principal focus (F).
- A ray directed towards the center of curvature (C) is reflected back along the same path.
- A ray directed towards the principal focus (F) reflects parallel to the principal axis.
Mirror Formula
The mirror formula is a mathematical relationship that relates the object distance (
), image distance (
), and the focal length (
) of a spherical mirror.
Mirror Formula:
![\[\displaystyle \boldsymbol{\frac{1}{f} = \frac{1}{v} + \frac{1}{u}}\]](https://deekshalearning.com/wp-content/ql-cache/quicklatex.com-7d5df4276b25690a2359a89f16e776c2_l3.png "Rendered by QuickLaTeX.com")
Where:
= Focal length
= Image distance- = Object distance
Sign Conventions:
- Distances measured in the direction of the incident light (towards the mirror) are taken as negative.
- Distances measured in the direction of the reflected light (away from the mirror) are taken as positive.
Magnification:
The magnification (m) produced by a mirror is the ratio of the height of the image (h′) to the height of the object (h):
![\[\displaystyle \boldsymbol{m = \frac{\textbf{h}'}{\textbf{h}} = \frac{-\textbf{v}}{\textbf{u}}}\]](https://deekshalearning.com/wp-content/ql-cache/quicklatex.com-87d4316c3f097332a4a962b9965cd8d3_l3.png "Rendered by QuickLaTeX.com")
- If
, the image is magnified (larger than the object). - If , the image is diminished (smaller than the object).
- If
, the image is the same size as the object.
, the image is magnified (larger than the object).
, the image is diminished (smaller than the object).
, the image is the same size as the object.Applications of Spherical Mirrors
Concave Mirrors:
- Used in Torches and Vehicle Headlights: Concave mirrors focus the light into a parallel beam, making them ideal for torches and headlights.
- Shaving or Makeup Mirrors: Concave mirrors magnify the image, making them useful for detailed tasks like shaving or applying makeup.
- Dentist Mirrors: Dentists use concave mirrors to get a magnified image of a patient’s teeth for better inspection.
Convex Mirrors:
- Rear-view Mirrors in Vehicles: Convex mirrors are used as rear-view mirrors because they provide a wider field of view, allowing drivers to see more area behind them.
- Security Mirrors in Stores: Convex mirrors are used in stores to allow shopkeepers to monitor larger areas.
Practice Questions with Answers
Q1: What is the principal focus of a concave mirror?
- Answer: The principal focus of a concave mirror is the point on the principal axis where parallel rays of light, after reflection from the mirror, converge.
Q2: A concave mirror has a focal length of 20 cm. Where should an object be placed in front of the mirror to obtain a real, inverted image of the same size as the object?
- Answer: The object should be placed at a distance equal to the radius of curvature, which is twice the focal length (R=2f). Hence, the object should be placed 40 cm in front of the mirror.
Q3: Why do we use convex mirrors as rear-view mirrors in vehicles?
- Answer: Convex mirrors are used as rear-view mirrors because they provide a wider field of view, allowing drivers to see more of the road behind them. They also form erect images, making them ideal for this purpose.
Q4: A convex mirror forms a virtual, erect image one-third the size of an object placed 12 cm in front of the mirror. Calculate the focal length of the mirror.
- Answer:
Given:
![\[\displaystyle \boldsymbol{m = \frac{1}{3}}, , \boldsymbol{u = -12} , \textbf{cm}, , \boldsymbol{v = ?}\]](https://deekshalearning.com/wp-content/ql-cache/quicklatex.com-d9d29b14d9a0acb4f6ec70e6f9e8b646_l3.png "Rendered by QuickLaTeX.com")
Since
![\[\displaystyle \boldsymbol{m = -\frac{v}{u}}\]](https://deekshalearning.com/wp-content/ql-cache/quicklatex.com-c0c339827e93ac21be4bd50546d4b74a_l3.png "Rendered by QuickLaTeX.com")
,
![\[\displaystyle \boldsymbol{\frac{1}{3} = \frac{-v}{-12}} \implies \boldsymbol{v = 4} , \textbf{cm}\]](https://deekshalearning.com/wp-content/ql-cache/quicklatex.com-ce42244565efd3f8a759b802d0646d52_l3.png "Rendered by QuickLaTeX.com")
Now, using the mirror formula:
![\[\displaystyle \boldsymbol{\frac{1}{f} = \frac{1}{4} + \frac{1}{-12} = \frac{3}{12} = \frac{1}{6}}\]](https://deekshalearning.com/wp-content/ql-cache/quicklatex.com-e48f6a9e3cff4de4a343c229c6bfa58c_l3.png "Rendered by QuickLaTeX.com")
Hence, 
.
FAQs
No, convex mirrors always form virtual, erect, and diminished images, as the reflected rays appear to diverge from a point behind the mirror.
Concave mirrors form real images when the object is placed beyond the focus. The reflected rays actually converge and meet at a point, forming a real image.
The image is virtual, erect, and magnified.
Related Topics
- Resistance Of A System Of Resistors
- Circuit Diagram
- Full Wave Rectifier
- Defects Of Vision And Their Correction
- Pressure
- Human Eye – Structure and Functioning
- Refraction Of Light Through A Prism
- Heating Effect Of Electric Current
- Physics FAQs
- Factors On Which The Resistance Of A Conductor Depends
- The Human Eye
- Atmospheric Refraction
- Acceleration
- Domestic Electric Circuits
- Faraday’s Law
Get Social