What is Bernoulli’s Principle?
Bernoulli’s principle asserts that the total mechanical energy of a moving fluid—comprising gravitational potential energy of elevation, fluid pressure energy, and kinetic energy of fluid motion—remains constant. This principle can be derived from the conservation of energy.
Bernoulli’s Principle Formula
Bernoulli’s equation relates pressure, kinetic energy, and gravitational potential energy of a fluid within a container. The formula is:
where:
p is the pressure exerted by the fluid,
v is the velocity of the fluid,
is the density of the fluid,
h is the height of the container.
Bernoulli’s equation provides valuable insight into the relationship between pressure, velocity, and elevation in fluid dynamics.
Derivation of Bernoulli’s Equation
Consider a pipe with varying diameter and height through which an incompressible fluid flows. The relationship between the cross-sectional areas (A), flow speed (v), height from the ground (y), and pressure (p) at two different points (1 and 2) is illustrated in the diagram below.
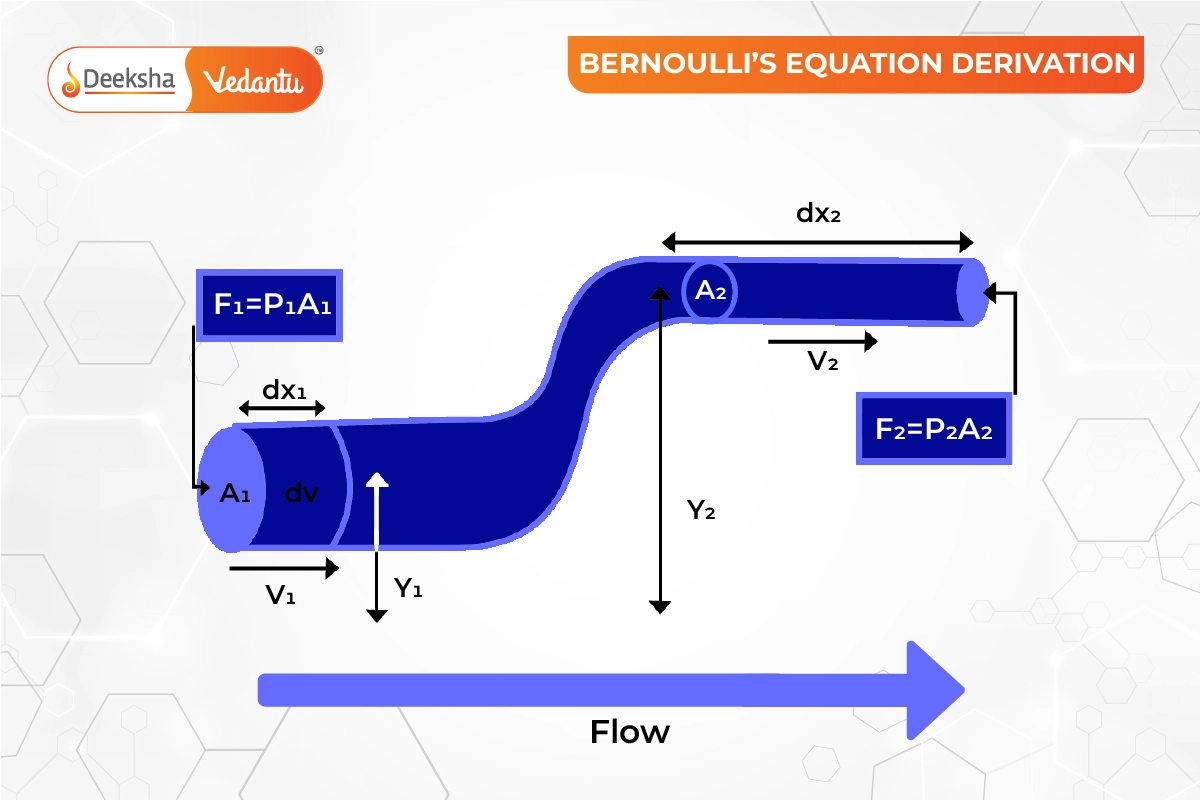
Assumptions:
The fluid density is constant at both points.
The fluid’s energy is conserved as there are no viscous forces.
The work done on the fluid is given by:
Since force F is pressure, p times area A:
The work done on the fluid is due to the change in gravitational potential energy and kinetic energy.
The change in kinetic energy (dK) is:
The change in potential energy (dU) is:
Thus, the energy equation becomes:
Substituting the expressions for dW, dK, and dU:
Dividing through by dv:
Rearranging this equation, we get Bernoulli’s equation:
This is Bernoulli’s equation, which demonstrates the conservation of energy for a flowing fluid.
Principle of Continuity
The principle of continuity states that for an incompressible fluid in a steady, streamline flow, the mass of fluid passing through different cross-sections of a pipe remains constant. In other words, the rate at which mass enters a section of the pipe is equal to the rate at which it exits.
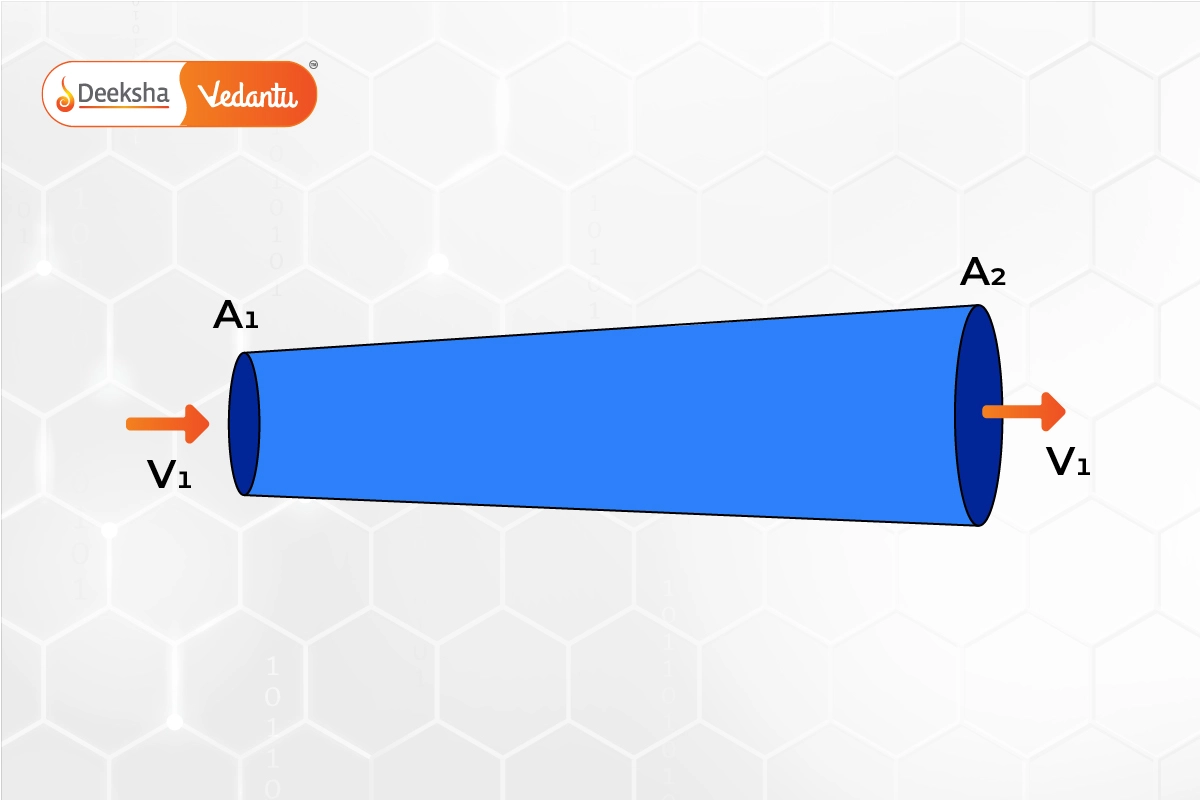
Mathematically, this can be expressed as:
Rate of mass entering = Rate of mass leaving
For a fluid flowing through a pipe with varying cross-sectional areas:
Rate of mass entering=ρA1V1Δt
Rate of mass leaving=ρA2V2Δt
Where:
ρ is the density of the fluid (constant for incompressible fluids)
A1 and A2 are the cross-sectional areas at points 1 and 2
V1 and V2 are the flow velocities at points 1 and 2
Δt is the time interval
Since the mass flow rate is conserved:
ρA1V1=ρA2V2
This is the Principle of Continuity, which shows that the product of cross-sectional area and velocity at any two points in a streamline flow is constant.
Applications of Bernoulli’s Principle and Equation
Bernoulli’s principle, which explains the relationship between the speed and pressure of a moving fluid, has several practical applications. It is especially useful in fields like fluid dynamics, oceanography, and acoustics. Here are some notable applications:
Venturi Meter:
- A device used to measure the flow rate of liquid through a pipe.
- It works by narrowing the pipe, which increases the fluid speed and decreases the pressure. This pressure difference is used to calculate the flow rate.
- The formula based on Bernoulli’s theorem is used to determine the flow rate through the Venturi meter.
Railway Station Phenomenon:
- When a train passes by at high speed, the air velocity between the train and a person increases, reducing the pressure. The higher pressure on the other side pushes the person towards the train.
- This can be explained using Bernoulli’s effect, which shows how increased speed leads to decreased pressure.
Relation Between Conservation of Energy and Bernoulli’s Equation
Bernoulli’s equation is derived from the conservation of energy principle applied to fluid flow. It states that the total mechanical energy (kinetic energy + potential energy + pressure energy) in a flowing fluid remains constant. This principle helps in understanding how changes in fluid speed and elevation affect pressure.
Bernoulli’s Equation at Constant Depth
When fluid flows at a constant depth (h1 = h2), Bernoulli’s equation simplifies to:
This shows the balance between pressure and kinetic energy.
Bernoulli’s Equation for Static Fluids
For static fluids where the velocity is zero (V1 = V2 = 0), Bernoulli’s equation becomes:
If we consider the second point to be at the reference level (h2 = 0), then:
This indicates the pressure difference due to height differences in a static fluid column
FAQs
Yes, a venturi meter uses Bernoulli’s principle to measure the flow rate of fluid through a pipe. As the fluid flows through a constricted section of the pipe, its speed increases and pressure decreases. The pressure difference is used to calculate the flow rate.
Bernoulli’s principle explains how lift is generated on an airplane wing. Airflow over the top of the wing moves faster than the airflow below, creating lower pressure above the wing and higher pressure below, resulting in an upward lift force.
The main assumptions for Bernoulli’s principle are:
- The fluid is incompressible.
- The fluid flow is steady.
- The fluid is non-viscous.
- The flow is along a streamline.
Bernoulli’s equation is derived from the principle of conservation of energy. For a flowing fluid, the total mechanical energy (comprising pressure energy, kinetic energy, and potential energy) remains constant along a streamline.
Bernoulli’s principle states that as the speed of a fluid increases, the pressure within the fluid decreases. This principle helps explain the behavior of fluids in motion and is fundamental in fluid dynamics.
Related Topics
- Pressure
- Magnetic Effects Of Electric Current
- Electric Potential And Potential Difference
- Concave Mirrors and Convex Mirrors
- Factors On Which The Resistance Of A Conductor Depends
- Faraday’s Law
- Magnet
- Zener Diode
- Force
- What is Hypothesis?
- Electricity
- Reflection Of Light
- Atmospheric Refraction
- Ohm’s Law
- Thermodynamics












Get Social