Trigonometry Formulas

In trigonometry, various formulas help solve problems involving trigonometric ratios (sin, cos, tan, sec, cosec, and cot), Pythagorean identities, and more. These formulas are essential for students in classes 10, 11, and 12 to score well in exams. Here is a simplified guide to the key trigonometric formulas.

Basic Trigonometric Function Formulas

In a right-angled triangle:

Hypotenuse: The longest side
Opposite side (Perpendicular): The side opposite to the angle
Adjacent side (Base): The side next to the angle

The six trigonometric functions are:

$\boldsymbol$

$\boldsymbol$

$\boldsymbol$

$\boldsymbol$

$\boldsymbol$

$\boldsymbol$

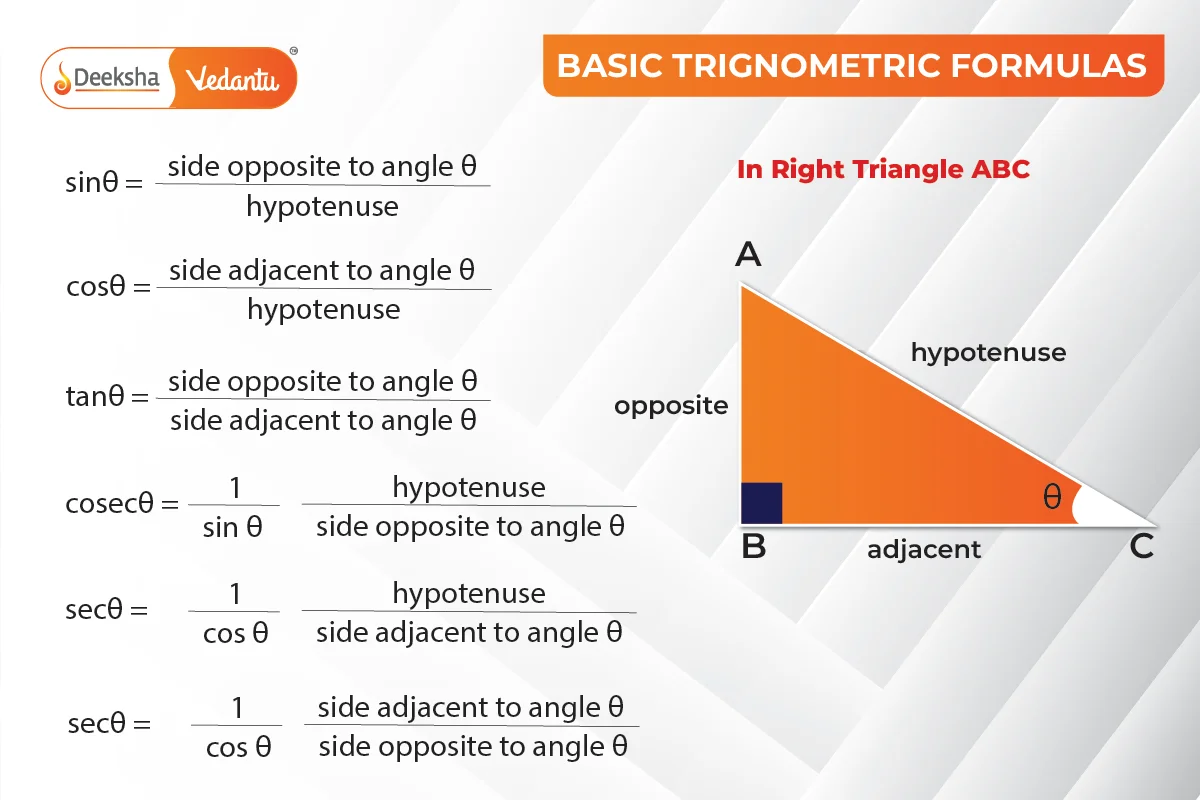
Reciprocal Identities

These identities express trigonometric functions in terms of their reciprocals:

$\boldsymbol$

$\boldsymbol$

$\boldsymbol$

$\boldsymbol$

$\boldsymbol$

$\boldsymbol$

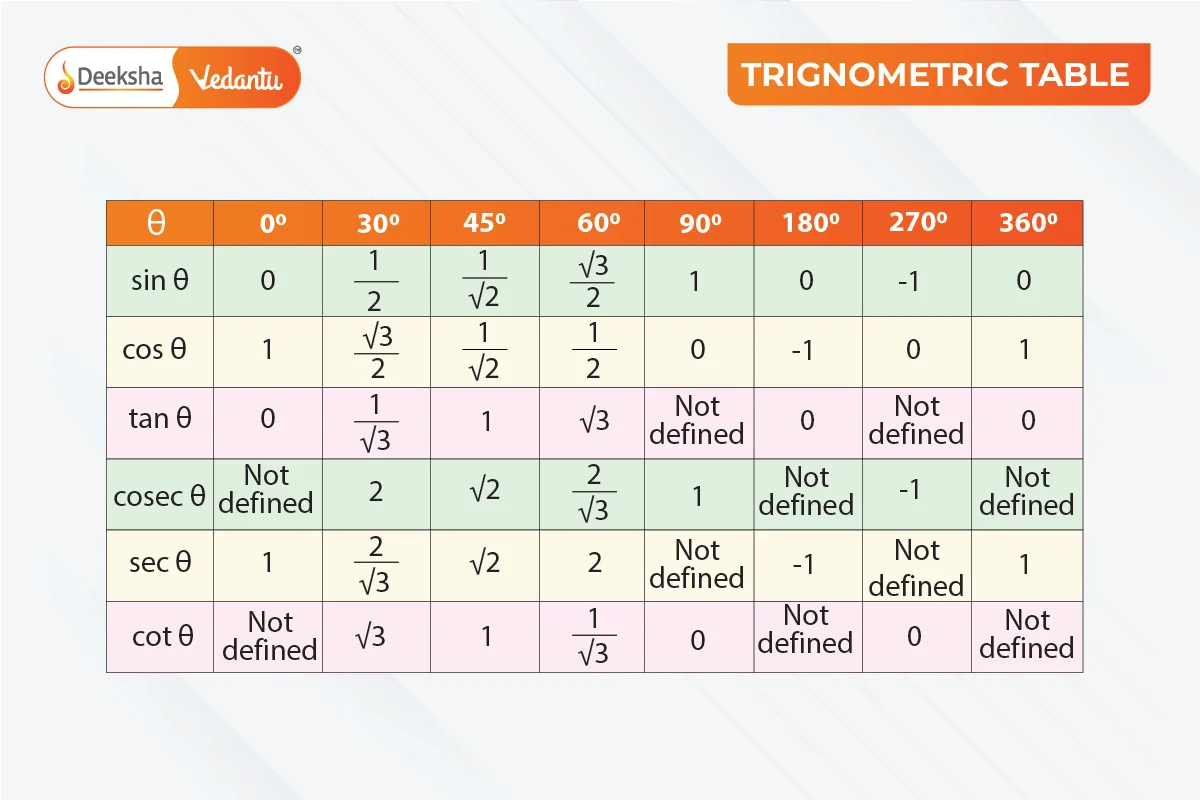
Trigonometry Table

$\boldsymbol$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol$
$\boldsymbol$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol$
$\boldsymbol$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol$
$\boldsymbol$	$\boldsymbol$	$\boldsymbol{\frac}$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol$
$\boldsymbol$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol{\frac}$	$\boldsymbol$
$\boldsymbol$	$\boldsymbol$	$\boldsymbol{\frac}$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol$
$\boldsymbol$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol$	$\boldsymbol{\frac}$	$\boldsymbol$

Periodicity Identities (in Radians)

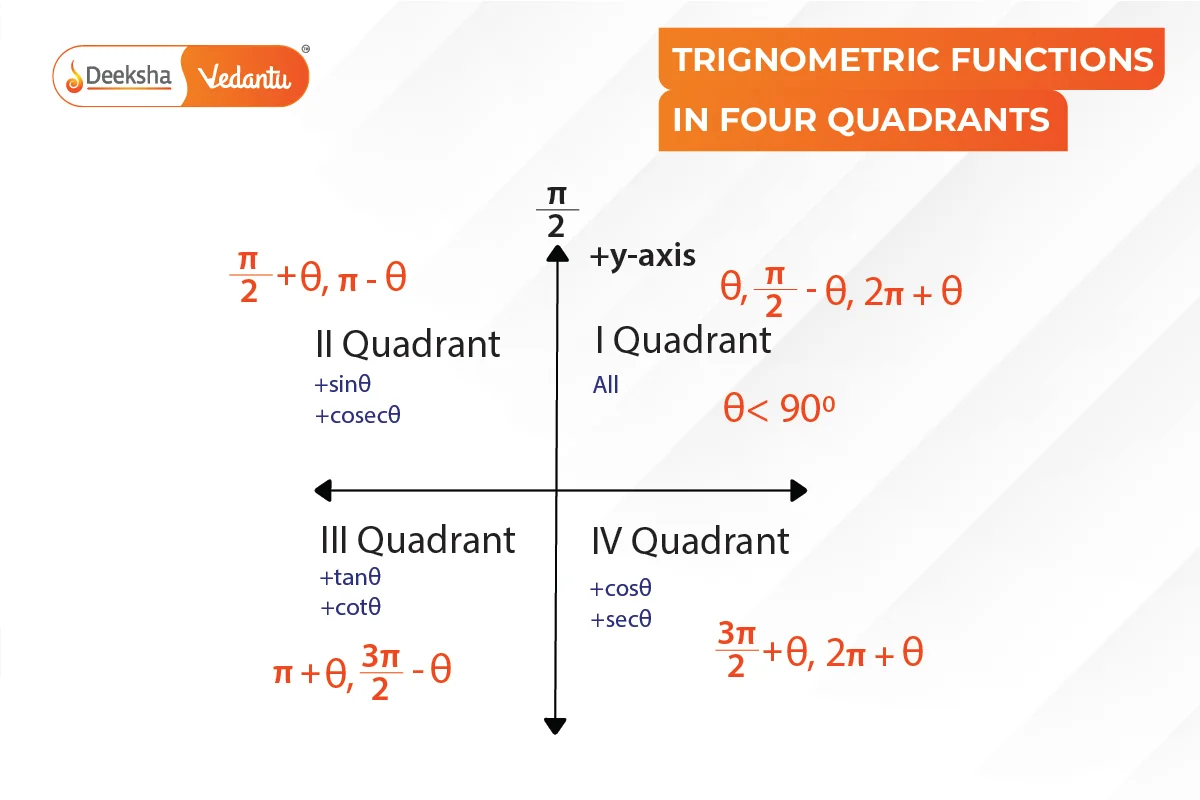
These identities shift angles by π/2, π, 2π, etc.:

$\boldsymbol$

$\boldsymbol$

$\boldsymbol$

$\boldsymbol$

Cofunction Identities (in Degrees)

These identities relate trigonometric functions of complementary angles:

$\boldsymbol$
$\boldsymbol$
$\boldsymbol$
$\boldsymbol$
$\boldsymbol$
$\boldsymbol$

Sum & Difference Identities

$\boldsymbol$
$\boldsymbol$
$\boldsymbol$
$\boldsymbol$

Double Angle Identities

$\boldsymbol$

Triple Angle Identities

$\boldsymbol$ \\
$\boldsymbol$

Half Angle Identities

$\boldsymbol$

$\boldsymbol$

Inverse Trigonometry Formulas

$\boldsymbol$
$\boldsymbol$
$\boldsymbol$
$\boldsymbol$
$\boldsymbol$
$\boldsymbol$

Trigonometry Formulas Major Systems

Trigonometric formulas are divided into two main categories:

Trigonometric Identities: These are true for all values of the variables.
Trigonometric Ratios: These relate the angles to the sides of a right-angled triangle.

These formulas are crucial for solving problems in trigonometry and understanding various applications in mathematics.

FAQs

What are double angle identities?admin2024-07-27T14:07:51+05:30

What are double angle identities?

Double angle identities express trigonometric functions of double angles:

$\boldsymbol$
$\boldsymbol$
$\boldsymbol$

What are sum and difference identities?admin2024-07-27T14:08:38+05:30

What are sum and difference identities?

Sum and difference identities are used to find the sine and cosine of the sum or difference of two angles:

$\boldsymbol$
$\boldsymbol$
$\boldsymbol$
$\boldsymbol$

What are the periodicity identities for trigonometric functions?admin2024-07-27T14:01:55+05:30

What are the periodicity identities for trigonometric functions?

Periodicity identities allow shifting angles by $\boldsymbol, \boldsymbol, \boldsymbol$ , etc.:

$\boldsymbol{\sin\left(\frac{2} - \theta\right) = \cos \theta}$
$\boldsymbol{\cos\left(\frac{2} - \theta\right) = \sin \theta}$
$\boldsymbol$
$\boldsymbol$

How do you find the sine and cosine of common angles?admin2024-07-27T14:05:47+05:30

How do you find the sine and cosine of common angles?

Angle	sin	cos
$\boldsymbol{0^\circ}$	$\boldsymbol{0}$	$\boldsymbol{1}$
$\boldsymbol{30^\circ}$	$\boldsymbol{\frac{1}{2}}$	$\boldsymbol{\frac{\sqrt{3}}{2}}$
$\boldsymbol{45^\circ}$	$\boldsymbol{\frac{1}{\sqrt{2}}}$	$\boldsymbol{\frac{1}{\sqrt{2}}}$
$\boldsymbol{60^\circ}$	$\boldsymbol{\frac{\sqrt{3}}{2}}$	$\boldsymbol{\frac{1}{2}}$
$\boldsymbol{90^\circ}$	$\boldsymbol{1}$	$\boldsymbol{0}$

What are reciprocal identities in trigonometry?admin2024-07-27T14:20:02+05:30

What are reciprocal identities in trigonometry?

Reciprocal identities relate trigonometric functions to their reciprocals:

$\boldsymbol$
$\boldsymbol$
$\boldsymbol$
$\boldsymbol$
$\boldsymbol$
$\boldsymbol$

What are the basic trigonometric functions?admin2024-07-27T14:51:31+05:30

What are the basic trigonometric functions?

The basic trigonometric functions are:

- $\boldsymbol$

- $\boldsymbol$

- $\boldsymbol$

- $\boldsymbol$

- $\boldsymbol$

- $\boldsymbol$

Basic Trigonometric Function Formulas

Reciprocal Identities

Trigonometry Table

Periodicity Identities (in Radians)

Cofunction Identities (in Degrees)

Sum & Difference Identities

Double Angle Identities

Triple Angle Identities

Half Angle Identities

Inverse Trigonometry Formulas

Trigonometry Formulas Major Systems

FAQs

Reciprocal identities relate trigonometric functions to their reciprocals:

Related Topics

Related Posts

Table of Contents

Join Deeksha Vedantu

> PU + Competitive Exam CoachingPreferred Choice For Toppers25+ Years of Academic Excellence70k+ Success Stories

Related Pages

Latest Posts

Top Links

Top Resources

Contact Us

Head Office

Get Social