Understanding Probability: The Mathematics of Chance
Probability is a mathematical concept that measures the likelihood of an event occurring. It ranges between 0 and 1, where 0 indicates an impossible event and 1 signifies a certain outcome. This concept is pivotal in predicting the chances of various outcomes in experiments or real-life situations.
What is Probability?
Probability quantifies the chance of an event happening. It’s calculated as the ratio of favorable outcomes to the total number of outcomes. For instance, when you flip a coin, the probability of getting heads is 1/2 since there are two possible outcomes and one favorable outcome.
Probability Formula
The basic probability formula is: P(E)=(Total number of outcomes)/(Number of favorable outcomes).
Examples of Probability Calculations:
- Picking a Yellow Pillow: With 3 red, 2 yellow, and 1 blue pillow, the probability of picking a yellow one is 2/6 or 1/3.
- Picking a Green Bottle: If 450 out of 1000 bottles are green, then the probability is 450/1000 = 0.45.
Probability Trees
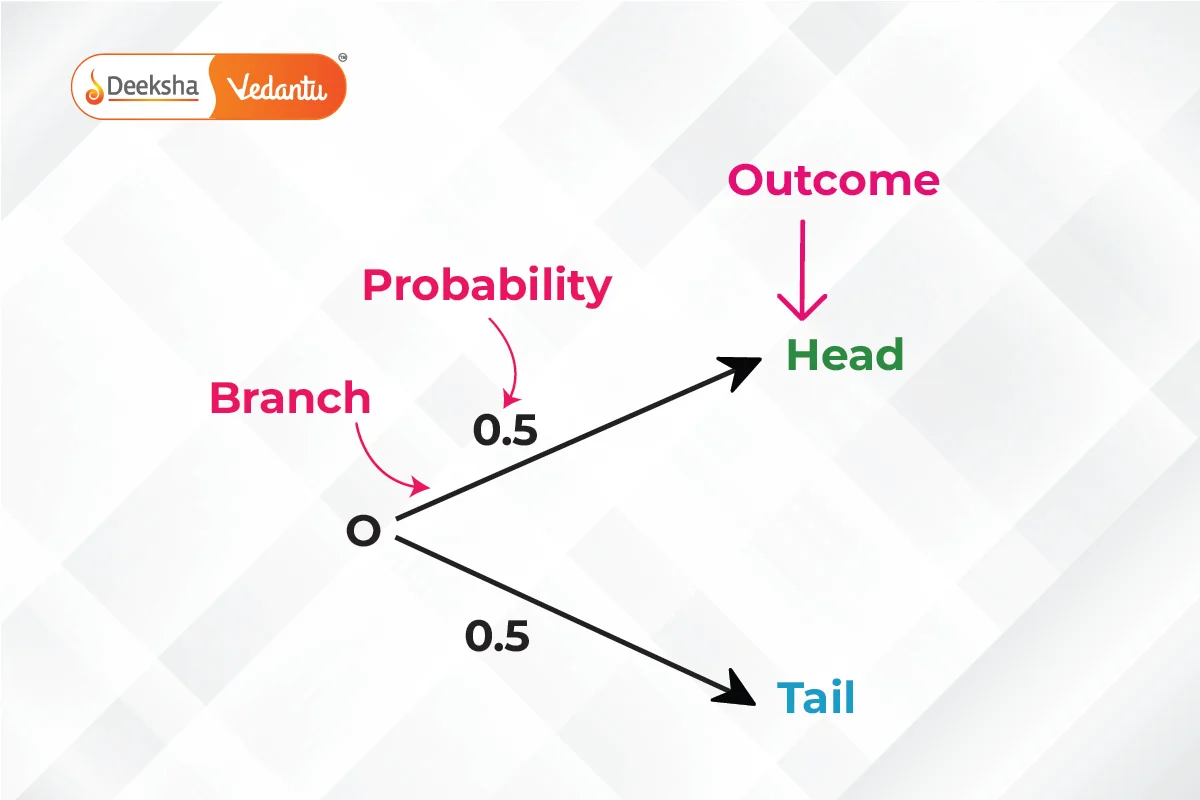
Probability trees help visualize and calculate the probabilities of sequential events. Each branch shows a possible outcome, and the ends represent the final outcomes, with the probability of each branch noted on the branch.
Types of Probability:
- Theoretical Probability: Based on possible outcomes. For example, flipping a coin has a theoretical probability of 1/2 for heads.
- Experimental Probability: Based on experimental results. For example, observing 6 heads in 10 coin tosses gives an experimental probability of 6/10 for heads.
- Axiomatic Probability: Based on set rules or axioms, applicable universally.
Solved Example on Probability:
If a container has 100 bottles with 45 green ones, the probability of drawing a green bottle is 45/100 = 0.45.
Probability Operations:
- Addition: To find the probability of either of two events happening.
- Multiplication: To find the probability of two independent events both occurring.
Conditional Probability
This measures the likelihood of an event given that another event has occurred. It helps in scenarios where the outcome of one event affects the outcome of another.
Complementary Events
These are events where the sum of their probabilities equals 1. For example, the probability of not drawing a heart from a deck of cards complements the probability of drawing a heart.
Probability and Real-Life Applications:
Probability theory assists in various fields, such as weather forecasting, risk assessment, sports, and more. It helps model different scenarios and predict outcomes effectively.
FAQs
Complementary events are two outcomes of an event that sum to a probability of 1, such as passing or failing a test.
No, probability values range from 0 to 1.
A common example is tossing a coin, where the probability of getting heads is 0.5.
Calculate probability using the formula P(E)=(Total number of outcomes)/(Number of favorable outcomes).
Probability measures the likelihood of an event occurring, ranging from 0 (impossible) to 1 (certain).





Get Social