The mean of grouped data is a statistical measure that represents the arithmetic average of a dataset divided into class intervals. This essential measure of central tendency helps summarize large amounts of data into a single representative value. It plays a vital role in analyzing, organizing, and interpreting grouped datasets effectively, especially in various real-world scenarios such as population studies, financial analysis, quality control in manufacturing, and scientific research. Understanding the mean allows statisticians and analysts to make informed decisions and draw meaningful conclusions from vast datasets.
The process of calculating the mean of grouped data involves several steps. Initially, the raw data must be systematically grouped into class intervals. Next, the midpoints (class marks) of each interval are identified, and the frequencies of data points in each class are noted. Using a specific formula tailored for grouped data, the mean is then determined, providing an efficient way to analyze larger datasets and identify central tendencies within organized numerical distributions.
Key Concepts for Mean of Grouped Data
- Class Intervals: These are specific ranges into which raw data is categorized, enabling better organization and interpretation of large datasets.
- Frequency (f): This represents the number of occurrences of data points within each class interval, indicating the concentration of data values.
- Class Mark (x): The midpoint of each class interval, calculated using the following formula:
- Cumulative Frequency: This shows the running total of frequencies up to a specific class interval, helping to determine medians and other statistical measures.
- Mean Formula for Grouped Data:
- Where:
= sum of the product of frequency and class mark
= total frequency
- Assumed Mean Method (Simplified Method):
- Used when dealing with large datasets, this method simplifies the calculations.
- Formula:
- Where:
= assumed mean (a value close to the actual mean, often chosen from one of the class marks)
= deviation of the class mark from the assumed mean (
)
= class width (difference between consecutive class intervals)
- Class Width: This is the difference between the upper and lower limits of a class interval, used to maintain uniform intervals in the dataset.
- Weighted Mean: If certain data classes carry more significance, their frequencies can be weighted to determine a more accurate mean.
Step-by-Step Example: Calculating the Mean of Grouped Data
Example: Calculate the mean of the following grouped data:
| Class Interval | Frequency (f) |
| 10-20 | 4 |
| 20-30 | 6 |
| 30-40 | 8 |
| 40-50 | 10 |
| 50-60 | 2 |
Solution:
- Find the class marks:
- For 10-20:
, for 20-30:
, for 30-40:
, for 40-50:
, and for 50-60:
.
- Multiply frequency by class mark:
| Class Interval | Frequency (f) | Class Mark (x) | |
| 10-20 | 4 | 15 | 60 |
| 20-30 | 6 | 25 | 150 |
| 30-40 | 8 | 35 | 280 |
| 40-50 | 10 | 45 | 450 |
| 50-60 | 2 | 55 | 110 |
- Calculate the mean:
Answer: The mean is .
Example Using Assumed Mean Method
Example: Calculate the mean using the assumed mean method for the following grouped data:
| Class Interval | Frequency (f) |
| 0-10 | 5 |
| 10-20 | 7 |
| 20-30 | 15 |
| 30-40 | 10 |
| 40-50 | 3 |
Solution:
- Assumed mean (a): Select
from the class interval 20-30 as the assumed mean.
- Calculate deviations:
| Class Interval | Frequency (f) | Class Mark (x) | ||
| 0-10 | 5 | 5 | -20 | -100 |
| 10-20 | 7 | 15 | -10 | -70 |
| 20-30 | 15 | 25 | 0 | 0 |
| 30-40 | 10 | 35 | 10 | 100 |
| 40-50 | 3 | 45 | 20 | 60 |
- Calculate mean:
Answer: The mean is .
Applications of Mean of Grouped Data
- Business Analytics: Helps organizations analyze revenue trends, employee productivity, and customer satisfaction.
- Economics: Assists in evaluating average income levels, inflation rates, and market growth trends.
- Healthcare: Enables the analysis of average patient recovery times, success rates of treatments, and healthcare facility performance.
- Education: Provides insights into student performance trends, attendance rates, and academic growth over time.
- Sports Analytics: Assists in tracking player performance metrics, game statistics, and predicting match outcomes based on historical data.
- Environmental Studies: Evaluates average temperatures, rainfall statistics, and pollution levels over time.



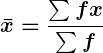
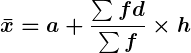
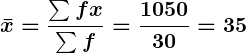
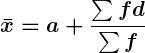


Get Social